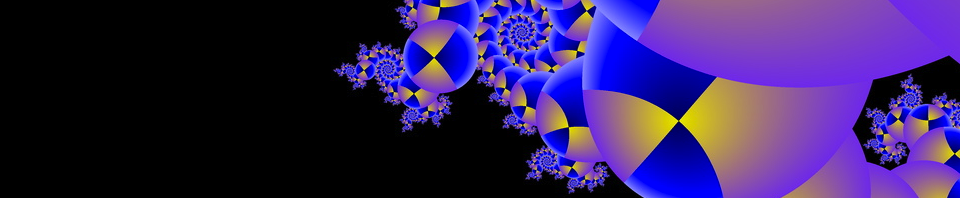
Portion of a Julia set based on the formula z/(z^2 + 1) + c, c = 0.06 – 0.02 i . It was generated with the “imaginary stalks” orbit trap option, and the stalks were mapped with two distinct color maps.
I’m quite fond of this one. I may rename it someday if I can think of a cleverer title, but “Droop” will do for now. I like the way it clearly illustrates the nature of fractal self-similarity. One can usually pick out by eye infinitely repeating progressions of (distorted) copies of an image in any fractal, but many images are too complicated to allow easy discernment of the truly complex nature of fractal self similarity. This fractal, due to its simplicity, allows us to see the way independent self-similar mappings can intertwine in a single image.
Notice how the image seems to be made up of a drooping pile of oval shapes. The components of the pile curl into a spiral at the top, as each component gets smaller in a clearly infinite progression.
However, every oval in itself contains an infinite series of concentric distorted ovals. In a depresion at the top of each distorted oval sits a complete copy of the entire image. The mappings that carries the image into each of the progression of smaller images inside the oval are entirely separate fromt the mapping described in the previous paragraph.